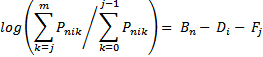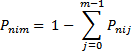Andrich RSM and polytomous PROX are difficult to estimate and explain to the non-technical. Meanwhile the Bradley-Massof polytomous pseudo-Rasch model is simpler - Bradley C., Massof R. (2018) Method of successive dichotomizations: An improved method for estimating measures of latent variables from rating scale data -. In the Andrich RSM, the threshold (category) parameters are determined by the relative frequencies of the category immediately above vs. the frequency of the category immediately below the threshold. In the BM model, the parameters are determined by the frequencies of all categories above vs. all categories below the threshold. The BM model makes the parameters immediately interpretable on the latent variable as category boundaries (unlike Andrich RSM). There are no disordered thresholds. Categories can be split or combined without altering the relationships between the other thresholds. In Andrich RSM, splitting or combining categories changes the relationships between all the thresholds.
The Andrich Rating-Scale Model, the Masters' Partial Credit Model and the Linacre Grouped-Rating-Scale Model can all be written in this way:
|
(1) |
where Fk indicates RSM, which can be written as Fik for PCM and Fgk for GRSM with Dgi where g indicates the item belongs to item-group g. The number of categories is m+1, and F0 = 0 or any convenient constant (it cancels out of the algebra).
Equation (1) hides the fundamental structure of these Rasch models, which is revealed when we rewrite this equation in logit-linear form:
|
(2) |
We can see here the simple additive (linear) form of a Rasch model so that each parameter has a "sufficient statistic" (= raw score or count in the data).
We can write the BM model in the same way conceptrually. This is not an exact algebraic representation of the DM mode. The Pnij values are inferred from the frequency of the categories, 0 to m, in the data.
|
(3) |
with the same options for Fj as RSM, PCM and GRSM. Then the DM thresholds correspond to a sequence of dichotomized items of equal or ascending difficulty, Fj >= Fj-1.
In practical terms, DM is the Samejima (1969) Graded Response Model with discrimination set to 1.0.
Pni0 is the probability of failing the easiest dichotomy.
|
(10) |
Pni1 is the probability of failing the second easiest dichotomy, but not failing the easiest dichotomy. This probability must be greater or equal to zero because the dichotomies are ordered.
|
(11) |
Pni2 is the probability of failing the third easiest dichotomy, but not failing the easier dichotomies,
|
(12) |
and so until the incremental probability of failing all the dichotomies is known. Then the probability of succeeding on the most difficult dichotomy, m, is the remaining probability Pnim.
|
(13) |
The reported person and item Standard Errors are the same as RSM.
See Example at Models-